Enable JavaScript to interact with content and submit forms on Wolfram websites. Learn how

WeaklyConnectedGraphComponents

WeaklyConnectedGraphComponents[g]

给出图 g 的弱连通分量.

WeaklyConnectedGraphComponents[g,{v₁,v₂,…}]

给出至少包含顶点 v₁、v₂、 … 之一的弱连通分量.

WeaklyConnectedGraphComponents[g,patt]

给出其中包含和模式 patt 相匹配的顶点的连通分量.

WeaklyConnectedGraphComponents[{vw,…},…]

用规则 vw 来指定图 g.

更多信息和选项

WeaklyConnectedComponents 返回 {c₁,c₂,…} 列表，其中以图给出每个分量 c_i.

如果有一系列的边连接 u 和 v，顶点 u 和 v 将都在同一个分量 c_i 中.
按长度为分量 c_i 排序，最大的分量排在第一位.
WeaklyConnectedGraphComponents 可用于无向图、有向图、多图和混合图.

范例

打开所有单元关闭所有单元

基本范例 (1)

给出图的弱连通分量：

突出显示分量：

范围 (8)

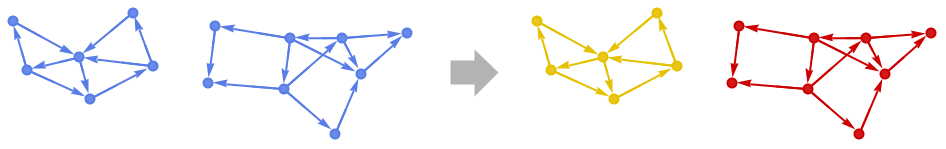
WeaklyConnectedGraphComponents 可用于无向图：

有向图：

多图：

混合图：

用规则来指定图：

选出含有至少一个指定顶点的弱连通图分量：

用模式来选出弱连通图分量的子集：

WeaklyConnectedGraphComponents 可用于大图：

应用 (1)

荷花池里的青蛙能跳到1.5英尺高以在25个荷叶之间跳跃. 根据荷叶的密度和 SpatialGraphDistribution 模拟青蛙的跳跃网络:

对任意荷花池进行抽样：

找出青蛙可在其中跳跃的最大范围的荷叶的数量：

求如果青蛙要到访每个荷叶，需要游水的次数：

属性和关系 (3)

对于无向图，连通分量和弱连通分量是相同的：

用 WeaklyConnectedGraphQ 来测试一个图是否为弱连通图：

弱连通图分量按顶点数量排序，最大的分量排在第一位：

顶部