WOLFRAM

Wolfram Language & System Documentation Center
Wolfram Language Home Page »

BlackmanHarrisWindow
✖
BlackmanHarrisWindow

✖

represents a Blackman–Harris window function of x.

Details

  • BlackmanHarrisWindow is a window function typically used in signal processing applications where data needs to be processed in short segments.
  • Window functions have a smoothing effect by gradually tapering data values to zero at the ends of each segment.
  • BlackmanHarrisWindow[x] is equal to  (48829 cos(2 pi x)+14128 cos(4 pi x)+1168 cos(6 pi x)+35875)/(100000) -1/2<=x<=1/2; 0 TemplateBox[{x}, Abs]>1/2; .
  • BlackmanHarrisWindow automatically threads over lists.

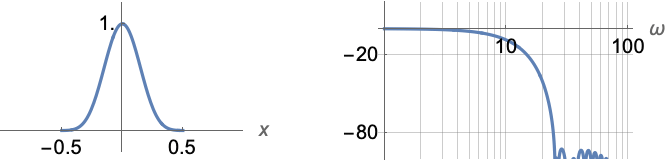
Examples

open allclose all

Basic Examples  (3)Summary of the most common use cases

Shape of a 1D Blackman–Harris window:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-55nvy5
Out[1]=1

Shape of a 2D Blackman–Harris window:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-yyuiog
Out[1]=1

Extract the continuous function representing the Blackman–Harris window:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-i5bqnh
Out[1]=1

Scope  (4)Survey of the scope of standard use cases

Evaluate numerically:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-rfdjbb
Out[1]=1

Translated and dilated Blackman–Harris window:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-fzqpy6
Out[1]=1

2D Blackman–Harris window with a circular support:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-h4a5s3
Out[1]=1

Discrete Blackman–Harris window of length 15:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-5yhlc9
Out[1]=1

Discrete 15×10 2D Blackman–Harris window:

In[2]:=2
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-ivcfcj
Out[2]=2

Applications  (3)Sample problems that can be solved with this function

Create a moving average filter of length 11:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-b4svbb
Out[1]=1

Taper the filter using a Blackman–Harris window:

In[2]:=2
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-1k06dy

Log-magnitude plot of the power spectra of the two filters:

In[3]:=3
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-65dzhv
Out[3]=3

Use a window specification to calculate sample PowerSpectralDensity:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-la7hx0

Calculate the spectrum:

In[2]:=2
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-xvpxo9

Compare to spectral density calculated without a windowing function:

In[3]:=3
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-012m6s
In[4]:=4
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-phnp33
Out[4]=4

The plot shows that window smooths the spectral density:

In[5]:=5
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-z97d2x
Out[5]=5

Compare to the theoretical spectral density of the process:

In[6]:=6
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-2bqb4v
Out[6]=6

Use a window specification for time series estimation:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-8tceex

Specify window for spectral estimator:

In[2]:=2
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-nkbyb7
Out[2]=2

Properties & Relations  (2)Properties of the function, and connections to other functions

The area under the Blackman–Harris window:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-bvk48s
Out[1]=1

Normalize to create a window with unit area:

In[2]:=2
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-uvswua
Out[2]=2

Fourier transform of the Blackman–Harris window:

In[1]:=1
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-hw628m
Out[1]=1

Power spectrum of the Blackman–Harris window:

In[2]:=2
✖
https://wolfram.com/xid/0bzrnjitc98rz6wlu-6mml6t
Out[2]=2
Wolfram Research (2012), BlackmanHarrisWindow, Wolfram Language function, https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html.
✖
Wolfram Research (2012), BlackmanHarrisWindow, Wolfram Language function, https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html.

Text

Wolfram Research (2012), BlackmanHarrisWindow, Wolfram Language function, https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html.

✖
Wolfram Research (2012), BlackmanHarrisWindow, Wolfram Language function, https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html.

CMS

Wolfram Language. 2012. "BlackmanHarrisWindow." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html.

✖
Wolfram Language. 2012. "BlackmanHarrisWindow." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html.

APA

Wolfram Language. (2012). BlackmanHarrisWindow. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html

✖
Wolfram Language. (2012). BlackmanHarrisWindow. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html

BibTeX

@misc{reference.wolfram_2025_blackmanharriswindow, author="Wolfram Research", title="{BlackmanHarrisWindow}", year="2012", howpublished="\url{https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html}", note=[Accessed: 13-May-2025 ]}

✖
@misc{reference.wolfram_2025_blackmanharriswindow, author="Wolfram Research", title="{BlackmanHarrisWindow}", year="2012", howpublished="\url{https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html}", note=[Accessed: 13-May-2025 ]}

BibLaTeX

@online{reference.wolfram_2025_blackmanharriswindow, organization={Wolfram Research}, title={BlackmanHarrisWindow}, year={2012}, url={https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html}, note=[Accessed: 13-May-2025 ]}

✖
@online{reference.wolfram_2025_blackmanharriswindow, organization={Wolfram Research}, title={BlackmanHarrisWindow}, year={2012}, url={https://reference.wolfram.com/language/ref/BlackmanHarrisWindow.html}, note=[Accessed: 13-May-2025 ]}