CoxIngersollRossProcess[μ,σ,θ,x0]
長期平均μ,ボラティリティ σ,調整速度θ,初期条件 x0のCox–Ingersoll–Ross過程を表す.


CoxIngersollRossProcess
CoxIngersollRossProcess[μ,σ,θ,x0]
長期平均μ,ボラティリティ σ,調整速度θ,初期条件 x0のCox–Ingersoll–Ross過程を表す.
詳細
- CoxIngersollRossProcessはCIR過程としても知られている.
- CoxIngersollRossProcessは,連続時間・連続状態のランダム過程である.
- Cox–Ingersoll–Ross過程の状態
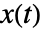 は伊藤微分方程式
は伊藤微分方程式 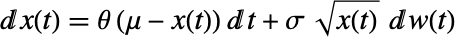 を満たす.ただし,
を満たす.ただし,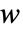 は標準WienerProcess[]に従う.
は標準WienerProcess[]に従う. - CoxIngersollRossProcessでは,x0は任意の正の実数.σ は任意の非零の実数,θ および μ は同じ符号の任意の非零の実数でよい.
- CoxIngersollRossProcessは,Mean,PDF,Probability,RandomFunction等の関数で使うことができる.
例題
すべて開く すべて閉じるスコープ (14)
基本的な用法 (9)
過程スライス特性 (5)
特性と関係 (3)
Cox–ingersoll–Ross過程は特殊なItoProcessである:
StratonovichProcessでもある:
関連するガイド
-
▪
- パラメトリック確率過程 ▪
- 確率微分方程式過程
テキスト
Wolfram Research (2012), CoxIngersollRossProcess, Wolfram言語関数, https://reference.wolfram.com/language/ref/CoxIngersollRossProcess.html.
CMS
Wolfram Language. 2012. "CoxIngersollRossProcess." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/CoxIngersollRossProcess.html.
APA
Wolfram Language. (2012). CoxIngersollRossProcess. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/CoxIngersollRossProcess.html
BibTeX
@misc{reference.wolfram_2025_coxingersollrossprocess, author="Wolfram Research", title="{CoxIngersollRossProcess}", year="2012", howpublished="\url{https://reference.wolfram.com/language/ref/CoxIngersollRossProcess.html}", note=[Accessed: 19-September-2025]}
BibLaTeX
@online{reference.wolfram_2025_coxingersollrossprocess, organization={Wolfram Research}, title={CoxIngersollRossProcess}, year={2012}, url={https://reference.wolfram.com/language/ref/CoxIngersollRossProcess.html}, note=[Accessed: 19-September-2025]}