MathieuCharacteristicExponent

MathieuCharacteristicExponent[a,q]

特性値 a とパラメータ q を持つマシュー(Mathieu)の奇関数に対し特性指数 r を与える．

詳細

  • 記号操作・数値操作の両方に適した数学関数である．
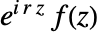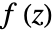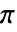  • マシュー関数はすべて の形を取る．ただし，は周期 を持つものとし，r はマシューの特性指数とする．
  • 特別な引数の場合，MathieuCharacteristicExponentは，自動的に厳密値を計算する．
  • MathieuCharacteristicExponentは任意の数値精度で評価できる．
  • MathieuCharacteristicExponentは自動的にリストに縫い込まれる．

例題

すべて開くすべて閉じる

例  (3)

数値的に評価する：

実数の部分集合上でプロットする：

複素数の部分集合上でプロットする：

スコープ  (15)

数値評価  (7)

数値的に評価する：

MathieuCharacteristicExponentは要素単位でリストに縫い込まれる：

高精度で評価する：

出力精度は入力精度に従う：

複素数入力：

高精度で効率的に評価する：

Aroundを使って平均的な場合の統計区間を計算する：

配列の要素ごとの値を計算する：

MatrixFunctionを使って行列のMathieuCharacteristicExponent関数を計算することもできる：

特定の値  (2)

簡単な厳密値は自動的に生成される：

MathieuCharacteristicExponent[3,q]=1.7となるような q の値を求める：

可視化  (3)

MathieuCharacteristicExponent関数を整数パラメータについてプロットする：

MathieuCharacteristicExponent関数を非整数パラメータについてプロットする：

MathieuCharacteristicExponentの実部をプロットする：

MathieuCharacteristicExponentの虚部をプロットする：

関数の特性  (3)

MathieuCharacteristicExponent[3,x]は非減少でも非増加でもない：

MathieuCharacteristicExponent[3,x]は非負でも非正でもない：

MathieuCharacteristicExponent[3,x]は凸でも凹でもない：

アプリケーション  (2)

周期ポテンシャルを持つシュレーディンガー(Schrödinger)方程式を解く：

ブロッホ(Bloch)の定理によると， がエネルギーバンドにあるとすると解には境界がある．エネルギーのギャップはMathieuCharacteristicExponentが消えることのない虚部を持つ の範囲に対応する：

次は，マシュー方程式の安定線図を示す：

特性と関係  (2)

特性指数関数と特性関数は互いに逆関数の関係にある：

プロットからMathieuCharacteristicExponent[x,0]=であることが分かる：

おもしろい例題  (1)

次は，周期ポテンシャルにおけるバンドギャップを示す：

Wolfram Research (1996), MathieuCharacteristicExponent, Wolfram言語関数， https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html.

テキスト

Wolfram Research (1996), MathieuCharacteristicExponent, Wolfram言語関数， https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html.

CMS

Wolfram Language. 1996. "MathieuCharacteristicExponent." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html.

APA

Wolfram Language. (1996). MathieuCharacteristicExponent. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html

BibTeX

@misc{reference.wolfram_2024_mathieucharacteristicexponent, author="Wolfram Research", title="{MathieuCharacteristicExponent}", year="1996", howpublished="\url{https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html}", note=[Accessed: 22-November-2024 ]}

BibLaTeX

@online{reference.wolfram_2024_mathieucharacteristicexponent, organization={Wolfram Research}, title={MathieuCharacteristicExponent}, year={1996}, url={https://reference.wolfram.com/language/ref/MathieuCharacteristicExponent.html}, note=[Accessed: 22-November-2024 ]}