MathieuGroupM12

MathieuGroupM12[]

represents the sporadic simple Mathieu group .

Details

  • By default, MathieuGroupM12[] is represented as a permutation group acting on points {1,…,12}.

Background & Context

  • MathieuGroupM12[] represents the Mathieu group 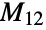, which is a group of order . It is one of the 26 sporadic simple groups of finite order. The default representation of MathieuGroupM12 is as a permutation group on the symbols 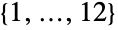having generators Cycles[{{1,4},{3,10},{5,11},{6,12}}] and Cycles[{{1,8,9},{2,3,4},{5,12,11},{6,10,7}}].
  • The Mathieu group 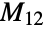is the second smallest of the sporadic finite simple groups. It was discovered (along with the other four Mathieu groups MathieuGroupM11, MathieuGroupM22, MathieuGroupM23 and MathieuGroupM24) by mathematician Émile Léonard Mathieu in the late 1800s, making these groups tied for first in chronological order of discovery among sporadic groups. MathieuGroupM12 is one of a very small number of groups to be sharply 5-transitive in the sense that there exists a unique group element 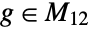mapping any unique 5-tuple 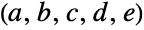of elements of MathieuGroupM12 to any other unique 4-tuple 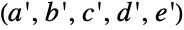therein. In addition to its permutation representation, 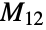can be defined in terms of generators and relations as . It may also generated by the permutations of the projective special linear group 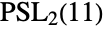together with the permutation Cycles[{{2,10},{3,4},{5,9},{6,7}}] via the identification of 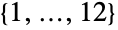with the projective line over the field 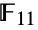of 11 elements. Along with the other sporadic simple groups, the Mathieu groups played a foundational role in the monumental (and complete) classification of finite simple groups.
  • The usual group theoretic functions may be applied to MathieuGroupM12[], including GroupOrder, GroupGenerators, GroupElements and so on. A number of precomputed properties of the Mathieu group 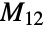are available via FiniteGroupData[{"Mathieu",12},"prop"].
  • MathieuGroupM12 is related to a number of other symbols. Along with MathieuGroupM11, MathieuGroupM22, MathieuGroupM23 and MathieuGroupM24, MathieuGroupM12 is one of five groups collectively referred to as the so-called "first generation" of sporadic finite simple groups. It is also one of 20 so-called "happy" sporadic groups, which all appear as a subquotient of the monster group.

Examples

open allclose all

Basic Examples  (1)

Order of the group :

Generators of a permutation representation of the group :

Properties & Relations  (1)

Separate group elements in different conjugacy classes:

These are the possible cyclic structures:

Corresponding orders (maximum is 11):

Number of elements in each class:

All together:

Wolfram Research (2010), MathieuGroupM12, Wolfram Language function, https://reference.wolfram.com/language/ref/MathieuGroupM12.html.

Text

Wolfram Research (2010), MathieuGroupM12, Wolfram Language function, https://reference.wolfram.com/language/ref/MathieuGroupM12.html.

CMS

Wolfram Language. 2010. "MathieuGroupM12." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/MathieuGroupM12.html.

APA

Wolfram Language. (2010). MathieuGroupM12. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/MathieuGroupM12.html

BibTeX

@misc{reference.wolfram_2024_mathieugroupm12, author="Wolfram Research", title="{MathieuGroupM12}", year="2010", howpublished="\url{https://reference.wolfram.com/language/ref/MathieuGroupM12.html}", note=[Accessed: 22-November-2024 ]}

BibLaTeX

@online{reference.wolfram_2024_mathieugroupm12, organization={Wolfram Research}, title={MathieuGroupM12}, year={2010}, url={https://reference.wolfram.com/language/ref/MathieuGroupM12.html}, note=[Accessed: 22-November-2024 ]}