ArithmeticGeometricMean
给出 a 和 b 的算术几何平均值.
更多信息
- 对某些特殊自变量,ArithmeticGeometricMean 自动计算其精确值.
- ArithmeticGeometricMean 可以计算到任意数值精度.
- ArithmeticGeometricMean 是关于 a 和 b 的齐次函数,在复平面
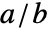 上有一个分支线,分支线从
上有一个分支线,分支线从 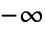 到 0.
到 0. - ArithmeticGeometricMean 自动逐项作用于列表.
- ArithmeticGeometricMean 可与 Interval 和 CenteredInterval 对象一起使用. »
范例
打开所有单元关闭所有单元范围 (25)
数值计算 (5)
用 Interval 和 CenteredInterval 对象计算最坏情况下的区间:
或用 Around 计算一般情况下的统计区间:
特殊值 (4)
可视化 (2)
函数属性 (10)
ArithmeticGeometricMean 可达到所有实数值:
ArithmeticGeometricMean 按元素线性作用于列表:
ArithmeticGeometricMean 并非解析函数:
TraditionalForm 格式化:
级数展开 (2)
应用 (4)
与 ArithmeticGeometricMean 进行比较:
用于计算算术几何平均值的迭代步骤的解析式,算术几何平均值用 ArithmeticGeometricMean 表示:
属性和关系 (3)
利用 FunctionExpand 展开 ArithmeticGeometricMean 成为其它函数:
证明 ArithmeticGeometricMean 满足一种超几何微分方程:
文本
Wolfram Research (1988),ArithmeticGeometricMean,Wolfram 语言函数,https://reference.wolfram.com/language/ref/ArithmeticGeometricMean.html (更新于 2022 年).
CMS
Wolfram 语言. 1988. "ArithmeticGeometricMean." Wolfram 语言与系统参考资料中心. Wolfram Research. 最新版本 2022. https://reference.wolfram.com/language/ref/ArithmeticGeometricMean.html.
APA
Wolfram 语言. (1988). ArithmeticGeometricMean. Wolfram 语言与系统参考资料中心. 追溯自 https://reference.wolfram.com/language/ref/ArithmeticGeometricMean.html 年