Series

Series[f,{x,x0,n}]

generates a power series expansion for f about the point x=x0 to order (x-x0)n, where n is an explicit integer.

Series[f,xx0]

generates the leading term of a power series expansion for f about the point x=x0.

Series[f,{x,x0,nx},{y,y0,ny},…]

successively finds series expansions with respect to x, then y, etc.

Details and Options

  • Series can construct standard Taylor series, as well as certain expansions involving negative powers, fractional powers, and logarithms.
  • Series detects certain essential singularities. On[Series::esss] makes Series generate a message in this case.
  • Series can expand about the point x=∞.
  • Series[f,{x,0,n}] constructs Taylor series for any function f according to the formula .
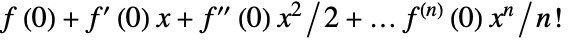  • Series effectively evaluates partial derivatives using D. It assumes that different variables are independent.
  • The result of Series is usually a SeriesData object, which you can manipulate with other functions.
  • Normal[series] truncates a power series and converts it to a normal expression.
  • SeriesCoefficient[series,n] finds the coefficient of the n^(th)-order term.
  • The following options can be given:
  • Analytic Truewhether to treat unrecognized functions as analytic
    Assumptions $Assumptionsassumptions to make about parameters
    SeriesTermGoalAutomaticnumber of terms in the approximation

Examples

open allclose all

Basic Examples  (4)

Power series for the exponential function around :

Convert to a normal expression:

Power series of an arbitrary function around :

In any operation on series, only appropriate terms are kept:

Find the leading term of a power series:

Scope  (10)

Univariate Series  (10)

Series can handle fractional powers and logarithms:

Symbolic parameters can often be used:

Laurent series with negative powers can be generated:

Truncate the series to the specified negative power:

Find power series for special functions:

Find the series for a function at a branch point:

With x assumed to be to the left of the branch point, a simpler result is given:

Piecewise functions:

Power series at infinity:

Series can give asymptotic series:

Series expansions of implicit solutions to equations:

Series expansions of unevaluated integrals:

Generalizations & Extensions  (4)

Power series in two variables:

Series is threaded element-wise over lists:

Series generates SeriesData expressions:

Series can work with approximate numbers:

Options  (4)

Analytic  (1)

Series by default assumes symbolic functions to be analytic:

Assumptions  (3)

Use Assumptions to specify regions in the complex plane where expansions should apply:

Without assumptions, piecewise functions appear:

Get expansions in Stokes regions:

Applications  (8)

Plot successive series approximations to :

Find a series expansion for a standard combinatorial problem:

Find Fibonacci numbers from a generating function:

Find Legendre polynomials by expanding a generating function:

Set up a generating function to enumerate ways to make change using U.S. coins:

The number of ways to make change for $1:

Find the lowest-order terms in a large polynomial:

Find higher-order terms in Newton's approximation for a root of f[x] near :

Plot the complex zeros for a series approximation to Exp[x]:

Properties & Relations  (10)

Series always only keeps terms up to the specified order:

Operations on series keep only the appropriate terms:

Normal converts to an ordinary polynomial:

Any mathematical function can be applied to a series:

Adding a series of lower order causes the higher-order terms to be dropped:

Differentiate a series:

Solve equations for series coefficients:

Find the list of coefficients in a series:

Use O[x] to force the construction of a series:

ComposeSeries treats a series as a function to apply to another series:

InverseSeries does series reversion to find the series for the inverse function of a series:

Use FunctionAnalytic to test whether a function is analytic:

An analytic function can be expressed as a Taylor series at each point of its domain:

The resulting polynomial approximates near 0:

Possible Issues  (7)

When there is an essential singularity, Series will attempt to factor it out:

Numeric values cannot be substituted directly for the expansion variable in a series:

Use Normal to get a normal expression in which the substitution can be done:

Series must be converted to normal expressions before being plotted:

Power series with different expansion points cannot be combined:

Not all series are represented by expressions with head SeriesData:

Some functions cannot be decomposed into series of power-like functions:

Series does not change expressions independent of the expansion variable:

Wolfram Research (1988), Series, Wolfram Language function, https://reference.wolfram.com/language/ref/Series.html (updated 2020).

Text

Wolfram Research (1988), Series, Wolfram Language function, https://reference.wolfram.com/language/ref/Series.html (updated 2020).

CMS

Wolfram Language. 1988. "Series." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2020. https://reference.wolfram.com/language/ref/Series.html.

APA

Wolfram Language. (1988). Series. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/Series.html

BibTeX

@misc{reference.wolfram_2024_series, author="Wolfram Research", title="{Series}", year="2020", howpublished="\url{https://reference.wolfram.com/language/ref/Series.html}", note=[Accessed: 22-November-2024 ]}

BibLaTeX

@online{reference.wolfram_2024_series, organization={Wolfram Research}, title={Series}, year={2020}, url={https://reference.wolfram.com/language/ref/Series.html}, note=[Accessed: 22-November-2024 ]}