WOLFRAM

Wolfram Language & System Documentation Center
Wolfram Language Home Page »

DesignMatrix
✖
DesignMatrix

✖
DesignMatrix[{{x11,x12,…,y1},{x21,x22,…,y2},…},{f1,f2,…},{x1,x2,…}]

constructs the design matrix for the linear model β0+β1 f1+β2 f2+….

Details and Options

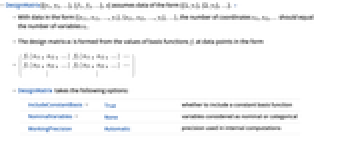
  • DesignMatrix[{y1,y2,…},{f1,f2,…},x] assumes data of the form {{1,y1},{2,y2},…}. »
  • With data in the form 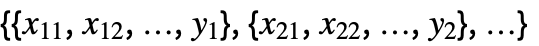{{x_(11),x_(12),...,y_(1)},{x_(21),x_(22),...,y_(2)},...}, the number of coordinates xi1, xi2, … should equal the number of variables xi.
  • The design matrix m is formed from the values of basis functions fi at data points in the form
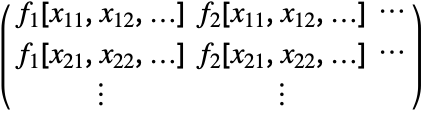
  • DesignMatrix takes the following options:
  • IncludeConstantBasis Truewhether to include a constant basis function
    NominalVariables Nonevariables considered as nominal or categorical
    WorkingPrecisionAutomaticprecision used in internal computations
  • With the setting IncludeConstantBasis->False, the design matrix for a model of form β1 f1+β2 f2+⋯ is constructed. »

Examples

open allclose all

Basic Examples  (3)Summary of the most common use cases

Design matrix for a linear model:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-c983bo
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-el342k

Add a quadratic term:

In[3]:=3
✖
https://wolfram.com/xid/0d6gptryqq-pdx4af

Leave out the constant term:

In[4]:=4
✖
https://wolfram.com/xid/0d6gptryqq-n0gkg

Design matrix with two predictor variables:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-cneqwe
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-f288qe

Include a product term:

In[3]:=3
✖
https://wolfram.com/xid/0d6gptryqq-gffoa2

Assume predictor values 1, 2, …:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-fyzmy7

Scope  (2)Survey of the scope of standard use cases

Use any numeric functions of the predictors:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-i7nkhf
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-ewjki4

Get the design matrix using exact arithmetic:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-b8bq5b
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-g80850

Use machine arithmetic:

In[3]:=3
✖
https://wolfram.com/xid/0d6gptryqq-dmlo52

Use arbitrary-precision arithmetic:

In[4]:=4
✖
https://wolfram.com/xid/0d6gptryqq-gxom8

Use fixed 24-digit precision arithmetic:

In[5]:=5
✖
https://wolfram.com/xid/0d6gptryqq-hv6cui

Options  (3)Common values & functionality for each option

IncludeConstantBasis  (1)

A constant term is included by default:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-eyj6fh
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-v0dl8

Construct a design matrix without a constant term:

In[3]:=3
✖
https://wolfram.com/xid/0d6gptryqq-mt4d6

NominalVariables  (2)

Treat x as a numeric variable:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-6jf3q
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-bcndyw

Treat x as nominal:

In[3]:=3
✖
https://wolfram.com/xid/0d6gptryqq-ga20p2

Use nominal variables that are not numeric:

In[4]:=4
✖
https://wolfram.com/xid/0d6gptryqq-ezwp9p

Treat only x as nominal:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-dvr0zy

Treat all predictors as nominal:

In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-bp7prx

Properties & Relations  (1)Properties of the function, and connections to other functions

DesignMatrix constructs the design matrix used by LinearModelFit:

In[1]:=1
✖
https://wolfram.com/xid/0d6gptryqq-iec2z9
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6gptryqq-9aa8q
In[3]:=3
✖
https://wolfram.com/xid/0d6gptryqq-jnxrgq
In[4]:=4
✖
https://wolfram.com/xid/0d6gptryqq-9ucpk

The matrix is the same for GeneralizedLinearModelFit:

In[5]:=5
✖
https://wolfram.com/xid/0d6gptryqq-dvgeq
In[6]:=6
✖
https://wolfram.com/xid/0d6gptryqq-fzifxk
Wolfram Research (2008), DesignMatrix, Wolfram Language function, https://reference.wolfram.com/language/ref/DesignMatrix.html.
✖
Wolfram Research (2008), DesignMatrix, Wolfram Language function, https://reference.wolfram.com/language/ref/DesignMatrix.html.

Text

Wolfram Research (2008), DesignMatrix, Wolfram Language function, https://reference.wolfram.com/language/ref/DesignMatrix.html.

✖
Wolfram Research (2008), DesignMatrix, Wolfram Language function, https://reference.wolfram.com/language/ref/DesignMatrix.html.

CMS

Wolfram Language. 2008. "DesignMatrix." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/DesignMatrix.html.

✖
Wolfram Language. 2008. "DesignMatrix." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/DesignMatrix.html.

APA

Wolfram Language. (2008). DesignMatrix. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/DesignMatrix.html

✖
Wolfram Language. (2008). DesignMatrix. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/DesignMatrix.html

BibTeX

@misc{reference.wolfram_2025_designmatrix, author="Wolfram Research", title="{DesignMatrix}", year="2008", howpublished="\url{https://reference.wolfram.com/language/ref/DesignMatrix.html}", note=[Accessed: 01-April-2025 ]}

✖
@misc{reference.wolfram_2025_designmatrix, author="Wolfram Research", title="{DesignMatrix}", year="2008", howpublished="\url{https://reference.wolfram.com/language/ref/DesignMatrix.html}", note=[Accessed: 01-April-2025 ]}

BibLaTeX

@online{reference.wolfram_2025_designmatrix, organization={Wolfram Research}, title={DesignMatrix}, year={2008}, url={https://reference.wolfram.com/language/ref/DesignMatrix.html}, note=[Accessed: 01-April-2025 ]}

✖
@online{reference.wolfram_2025_designmatrix, organization={Wolfram Research}, title={DesignMatrix}, year={2008}, url={https://reference.wolfram.com/language/ref/DesignMatrix.html}, note=[Accessed: 01-April-2025 ]}