ONanGroupON
表示散在 O'Nan 单群 
.
背景
- ONanGroupON[] 表示 O'Nan 群
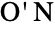，是阶数为 ![TemplateBox[{2, 9}, Superscript].TemplateBox[{3, 4}, Superscript].5.TemplateBox[{7, 3}, Superscript].11.19.31](Files/ONanGroupON.zh/3.png "TemplateBox[{2, 9}, Superscript].TemplateBox[{3, 4}, Superscript].5.TemplateBox[{7, 3}, Superscript].11.19.31")
的群. 是 26 个散在有限单群之一，有时也被称作 O'Nan–Sims 群. - O'Nan 群
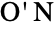是第十三大的散在有限单群. 由数学家 Michael O'Nan 发现，并由 Charles Sims 于二十世纪七十年代中期明确构建. ONanGroupON 是在研究所谓的具有 Alperin 型 Sylow 2 子群的群时被首次发现的. O'Nan 群有一个在 
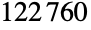个符号上的置换表示，有一对在有七个元素的域 
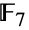上的 45 维模表示法的三重覆盖，还有许多极大子群，包括 Janko 群 
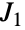、Mathieu 群 
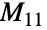和交错群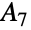 
. 与其他散在单群一起，
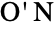在有限单群的重要（和完全）分类中发挥了基础性作用. - 常见的群论函数都可用于 ONanGroupON[]，包括 GroupOrder、GroupGenerators、GroupElements 等等. 然而，尽管 ONanGroupON[] 是一个置换群，因为其阶数较大，明确的置换表示对于直接实现是不切实际的. 所以，对其应用有些群论函数时，可能无法计算. 可通过 FiniteGroupData["ONan","prop"] 获取一些已预先算好的 O'Nan 群的属性.
- ONanGroupON 与其他一些符号有关. 与 JankoGroupJ1、JankoGroupJ3、JankoGroupJ4、LyonsGroupLy 和 RudvalisGroupRu 一起，ONanGroupON 是被称作贱民群 ("pariahs") 的六个散在单群之一 ，因为它们都不是魔群的子商.
范例
Wolfram Research (2010)，ONanGroupON，Wolfram 语言函数，https://reference.wolfram.com/language/ref/ONanGroupON.html.
文本
Wolfram Research (2010)，ONanGroupON，Wolfram 语言函数，https://reference.wolfram.com/language/ref/ONanGroupON.html.
CMS
Wolfram 语言. 2010. "ONanGroupON." Wolfram 语言与系统参考资料中心. Wolfram Research. https://reference.wolfram.com/language/ref/ONanGroupON.html.
APA
Wolfram 语言. (2010). ONanGroupON. Wolfram 语言与系统参考资料中心. 追溯自 https://reference.wolfram.com/language/ref/ONanGroupON.html 年