ParabolicCylinderD

ParabolicCylinderD[ν,z]

放物柱関数 TemplateBox[{nu, z}, ParabolicCylinderD]を与える．

詳細

  • 記号操作・数値操作の両方に適した数学関数である．
  • TemplateBox[{nu, z}, ParabolicCylinderD]は，ウェーバーの微分方程式 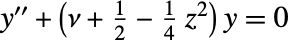を満足する．
  • 特別な引数の場合，ParabolicCylinderDは，自動的に厳密値を計算する．
  • ParabolicCylinderDは任意の数値精度で評価できる．
  • ParabolicCylinderDは自動的にリストに並列的な関数の適用を行う．
  • ParabolicCylinderD[ν,z]は，不連続な分枝切断線を持たない z の整関数である．
  • ParabolicCylinderDはIntervalオブジェクトおよびCenteredIntervalオブジェクトに使うことができる． »

例題

すべて開くすべて閉じる

例  (5)

数値的に評価する：

実数の部分集合上で をプロットする：

複素数の部分集合上でプロットする：

原点における級数展開：

Infinityにおける級数展開：

スコープ  (36)

数値評価  (7)

数値的に評価する：

高精度で評価する：

出力精度は入力精度に従う：

複素数入力：

高精度で効率よく評価する：

ParabolicCylinderDはIntervalオブジェクトおよびCenteredIntervalオブジェクトに使うことができる：

Aroundを使って平均的な場合の統計区間を計算する：

配列の要素ごとの値を計算する：

MatrixFunctionを使って行列のParabolicCylinderD関数を計算することもできる：

特定の値  (5)

記号的なパラメータについてのParabolicCylinderD：

ゼロにおける値：

無限大における極限値：

ParabolicCylinderDの最初の正の最大値を求める：

半整数パラメータで評価する：

可視化  (4)

整数()と半整数()の次数でParabolicCylinderD関数をプロットする：

の実部をプロットする：

の虚部をプロットする：

2つのパラメータの実部が変化する様子をプロットする：

第2種および第3種のParabolicCylinderD関数は異なる分岐構造を持つ：

関数の特性  (10)

ParabolicCylinderDはすべての実数値と複素値について定義される：

ParabolicCylinderDは要素単位でリストに縫い込まれる：

TemplateBox[{v, x}, ParabolicCylinderD]は の解析関数である：

TemplateBox[{n, x}, ParabolicCylinderD]は のとき非減少でも非増加でもない：

TemplateBox[{n, x}, ParabolicCylinderD]は のとき単射ではない：

ParabolicCylinderDは全射ではない：

TemplateBox[{n, x}, ParabolicCylinderD]は のとき非負でも非正でもない：

ParabolicCylinderDは特異点も不連続点も持たない：

TemplateBox[{n, x}, ParabolicCylinderD]は のとき凸でも凹でもない：

TraditionalFormによる表示：

微分  (3)

z についての一次導関数：

z についての高次導関数：

ν=2のとき，z についての高次導関数をプロットする：

z についての 次導関数の式：

級数展開  (5)

Seriesを使ってテイラー(Taylor)展開を求める：

の周りの最初の3つの近似をプロットする：

SeriesCoefficientを使った級数展開における一般項：

Infinityにおける級数展開を求める：

任意の記号方向 についての級数展開を求める：

生成点におけるテイラー展開：

関数の恒等式と簡約  (2)

関数の恒等式：

漸化恒等式：

一般化と拡張  (2)

記号的な第1引数の級数展開：

無限大における級数展開：

アプリケーション  (2)

任意のエネルギーについての二次振動子のシュレーディンガー(Schrödinger)方程式の解を求める：

ParabolicCylinderDはWeber方程式を解く：

特性と関係  (5)

FunctionExpandを使って ParabolicCylinderDを他の関数に展開する：

ParabolicCylinderDを含む式を積分する：

ParabolicCylinderDはDifferentialRootとして表すことができる：

ParabolicCylinderDはDifferenceRootとして表すことができる：

ParabolicCylinderDの指数母関数：

Wolfram Research (2007), ParabolicCylinderD, Wolfram言語関数， https://reference.wolfram.com/language/ref/ParabolicCylinderD.html.

テキスト

Wolfram Research (2007), ParabolicCylinderD, Wolfram言語関数， https://reference.wolfram.com/language/ref/ParabolicCylinderD.html.

CMS

Wolfram Language. 2007. "ParabolicCylinderD." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/ParabolicCylinderD.html.

APA

Wolfram Language. (2007). ParabolicCylinderD. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/ParabolicCylinderD.html

BibTeX

@misc{reference.wolfram_2024_paraboliccylinderd, author="Wolfram Research", title="{ParabolicCylinderD}", year="2007", howpublished="\url{https://reference.wolfram.com/language/ref/ParabolicCylinderD.html}", note=[Accessed: 22-November-2024 ]}

BibLaTeX

@online{reference.wolfram_2024_paraboliccylinderd, organization={Wolfram Research}, title={ParabolicCylinderD}, year={2007}, url={https://reference.wolfram.com/language/ref/ParabolicCylinderD.html}, note=[Accessed: 22-November-2024 ]}