WOLFRAM

Wolfram Language & System Documentation Center
Wolfram Language Home Page »

RegionDimension
✖
RegionDimension

✖

gives the geometric dimension of the region reg.

Details and Options

  • The geometric dimension d of reg is the largest d such that a d-dimensional ball can be completely embedded in the region.
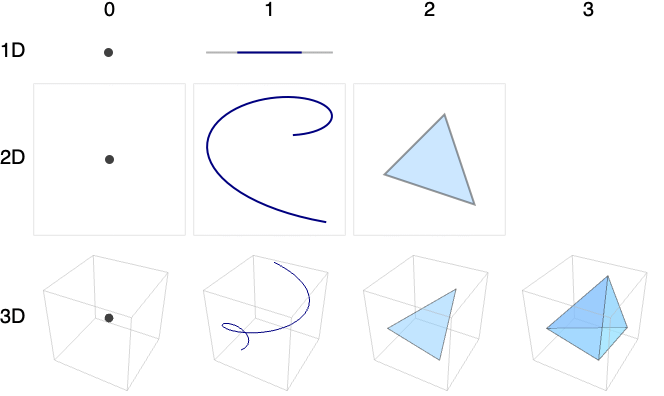
  • Typical names for regions of different dimensions include:
  • 0points
    1lines, curves, arcs, segments, intervals
    2planes, surfaces
    3solids, volumes
  • Example cases with rows corresponding to embedding dimension and columns to RegionDimension:
  • RegionDimension takes an Assumptions option that can be used to specify assumptions on parameters.

Examples

open allclose all

Basic Examples  (4)Summary of the most common use cases

The dimension of regions in :

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-kmy52s
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-fu7fny
Out[2]=2

The dimension of regions in :

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-b64xxx
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-p0rmz
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-c9f3le
Out[3]=3

The dimension of regions in :

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-cf7hvq
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-9572i
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-g218v8
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-cydx0v
Out[4]=4

The dimension of regions in :

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-bi05hm
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-bza0j1
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-igx9q
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-bc2s9n
Out[4]=4
In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-hr0a48
Out[5]=5

Scope  (17)Survey of the scope of standard use cases

Special Regions  (4)

Regions in including Point:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-cgwczk
Out[1]=1

Interval:

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-dj73or
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-eoamtr
Out[4]=4

Regions in including Point:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-ff95qq
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-c9j8dd
Out[2]=2

Line:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-bjgri3
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-cvkhkk
Out[4]=4

Circle:

In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-kyyvln
Out[5]=5
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-ca5l2m
Out[6]=6

Disk:

In[7]:=7
✖
https://wolfram.com/xid/0d6g6qvxrm-b1cbms
Out[7]=7
In[8]:=8
✖
https://wolfram.com/xid/0d6g6qvxrm-cdv8fs
Out[8]=8

Regions in including Point:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-hnubnh
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-eeut6h
Out[2]=2

Line:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-hoqrd8
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-mkjlej
Out[4]=4

Polygon:

In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-brf9tr
Out[5]=5
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-ln36yy
Out[6]=6

Cylinder:

In[7]:=7
✖
https://wolfram.com/xid/0d6g6qvxrm-c3xs9v
Out[7]=7
In[8]:=8
✖
https://wolfram.com/xid/0d6g6qvxrm-u9k2e
Out[8]=8

Regions in including Simplex in :

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-kukhfy
Out[1]=1

Cuboid in :

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-kojw4f
Out[2]=2

Ball in :

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-hhrdfi
Out[3]=3

Formula Regions  (3)

The dimension of a disk represented as an ImplicitRegion:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-bxcpan
In[10]:=10
✖
https://wolfram.com/xid/0d6g6qvxrm-dotrh7
Out[10]=10
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-qjnxx
Out[4]=4

A cylinder:

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-b9ijej
Out[2]=2

The dimension of a disk represented as a ParametricRegion:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-jgtmd
Out[1]=1

Using a rational parametrization of the disk:

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-d5r1up
Out[2]=2

A cylinder:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-mx0s
Out[3]=3

ImplicitRegion can have several components of different dimension:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-d4mmo3
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-irlsf
Out[2]=2

RegionDimension gives the largest dimension:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-bynuh2
Out[3]=3

Mesh Regions  (4)

The dimension of a BoundaryMeshRegion:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-dd1gl
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-if42fs
Out[2]=2

In 2D:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-d6f2da
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-dmxirt
Out[4]=4

In 3D:

In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-hc2qlx
Out[5]=5
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-b14w9
Out[6]=6

The dimension of a MeshRegion:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-ekd12c
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-c2bfdx
Out[2]=2

A 3D mesh in 3D:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-ecrmly
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-b52xw8
Out[4]=4

A 1D mesh embedded in 2D:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-qkprr
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-h9ete0
Out[2]=2

A MeshRegion can have components of different dimension:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-cuq63
Out[1]=1

The RegionDimension is the largest dimension:

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-8w1ev
Out[2]=2

Derived Regions  (4)

The dimension of a RegionIntersection:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-plli4y
In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-irl1hp
Out[5]=5
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-j0fjs3
Out[6]=6

The dimension of a TransformedRegion:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-f0ggnh
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-narsqv
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-exycnl
Out[3]=3

The dimension of a RegionBoundary:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-hgc525
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-emf8i2
Out[2]=2

RegionBoundary for a full-dimensional region is less than the original dimension:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-y259m
Out[3]=3

RegionDimension for an intersection can be less than the original dimensions:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-dpd69u
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-fmu1bx
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-f846ns
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-c4t094
Out[4]=4

The dimension can drop by more than one:

In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-bbv3v5
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-c61td
In[7]:=7
✖
https://wolfram.com/xid/0d6g6qvxrm-gx6mzl
Out[7]=7
In[8]:=8
✖
https://wolfram.com/xid/0d6g6qvxrm-bxtrwb
Out[8]=8

Geographic Regions  (2)

Polygons with GeoPosition:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-1lk1dy
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-8vjhh8
Out[2]=2

Polygons with GeoPositionXYZ:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-4dy58p
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-pzmsx0
Out[4]=4

Polygons with GeoPositionENU:

In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-sz3fv8
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-pjpdg1
Out[6]=6

Polygons with GeoGridPosition:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-ipsiqu
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-yjciq7
Out[2]=2

Applications  (8)Sample problems that can be solved with this function

A zero-dimensional object is a collection of points:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-fbzabv
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-dcyt69
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-ggfam6
Out[4]=4

A one-dimensional object is a collection of curves:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-s2wl0i
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-2fb88r
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-6dkko1
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-nhfz24
Out[4]=4
In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-7pz529
Out[5]=5

A two-dimensional object is a collection of surfaces:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-o3c6r2
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-r4aax9
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-tr5853
Out[3]=3

Use RegionDimension to tell the difference between a volume and a surface:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-o96zmr

Regions may be visually identical:

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-l80lle
Out[2]=2

But differ in dimensionality:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-c0rx0x
Out[3]=3

Compute dimension of regions that cannot be visualized:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-1t8fiv
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-qgogjo
Out[2]=2

The unit for RegionMeasure is with the length unit and :

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-jawpve
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-6kx2la

Compute the measure of each region:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-kz2mcn
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-lxkn5y
Out[4]=4

Compare with the result from RegionMeasure:

In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-r212h4
Out[5]=5

Extract MeshRegion primitives by dimension using MeshPrimitives:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-umzmd9
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-wl597j
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-fwf50r
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-xqnp7q
Out[4]=4

Select only full-dimensional primitives:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-5n771t
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-6npg2s
Out[2]=2

Properties & Relations  (8)Properties of the function, and connections to other functions

RegionDimension gives the largest dimension among parts of varying dimension:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-bhe75v
Out[1]=1

The RegionDimension is the largest dimension:

In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-4tces7
Out[2]=2

RegionEmbeddingDimension is the dimension of the space in which a region exists:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-63glw
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-kij29y
Out[2]=2

It is always greater than or equal to RegionDimension:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-34818
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-cs3bsw
Out[4]=4

DimensionalMeshComponents separates a mesh in different dimensional parts:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-qtw7p5
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-pa78u8
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-fsr3nj
Out[3]=3

RegionMeasure and RegionCentroid are dimension dependent:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-f2v8oh
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-bbsag0
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-d0al66
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-nzcwx
Out[4]=4

Integration over a region is dimension dependent:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-qvg69
Out[1]=1
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-euzy61
Out[2]=2

Since the dimension is 2, integration corresponds to a surface integral:

In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-d6qe7h
Out[3]=3
In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-kcghye
Out[4]=4

The RegionDimension of a RegionBoundary is one less than that of the input:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-cv2808
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-kkfx6u
Out[2]=2

The RegionDimension of a RegionUnion is equal to the largest input dimension:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-dn4uoh
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-kqq2h4
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-nhml0b
Out[3]=3

RegionDimension of a RegionIntersection is no larger than the smallest input dimension:

In[1]:=1
✖
https://wolfram.com/xid/0d6g6qvxrm-imfdq4
In[2]:=2
✖
https://wolfram.com/xid/0d6g6qvxrm-d8m3d0
Out[2]=2
In[3]:=3
✖
https://wolfram.com/xid/0d6g6qvxrm-1ny81
Out[3]=3

But it can be smaller:

In[4]:=4
✖
https://wolfram.com/xid/0d6g6qvxrm-jzyk63
In[5]:=5
✖
https://wolfram.com/xid/0d6g6qvxrm-lr3tgw
Out[5]=5
In[6]:=6
✖
https://wolfram.com/xid/0d6g6qvxrm-bwgo5l
Out[6]=6
Wolfram Research (2014), RegionDimension, Wolfram Language function, https://reference.wolfram.com/language/ref/RegionDimension.html.
✖
Wolfram Research (2014), RegionDimension, Wolfram Language function, https://reference.wolfram.com/language/ref/RegionDimension.html.

Text

Wolfram Research (2014), RegionDimension, Wolfram Language function, https://reference.wolfram.com/language/ref/RegionDimension.html.

✖
Wolfram Research (2014), RegionDimension, Wolfram Language function, https://reference.wolfram.com/language/ref/RegionDimension.html.

CMS

Wolfram Language. 2014. "RegionDimension." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/RegionDimension.html.

✖
Wolfram Language. 2014. "RegionDimension." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/RegionDimension.html.

APA

Wolfram Language. (2014). RegionDimension. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/RegionDimension.html

✖
Wolfram Language. (2014). RegionDimension. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/RegionDimension.html

BibTeX

@misc{reference.wolfram_2025_regiondimension, author="Wolfram Research", title="{RegionDimension}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/RegionDimension.html}", note=[Accessed: 19-May-2025 ]}

✖
@misc{reference.wolfram_2025_regiondimension, author="Wolfram Research", title="{RegionDimension}", year="2014", howpublished="\url{https://reference.wolfram.com/language/ref/RegionDimension.html}", note=[Accessed: 19-May-2025 ]}

BibLaTeX

@online{reference.wolfram_2025_regiondimension, organization={Wolfram Research}, title={RegionDimension}, year={2014}, url={https://reference.wolfram.com/language/ref/RegionDimension.html}, note=[Accessed: 19-May-2025 ]}

✖
@online{reference.wolfram_2025_regiondimension, organization={Wolfram Research}, title={RegionDimension}, year={2014}, url={https://reference.wolfram.com/language/ref/RegionDimension.html}, note=[Accessed: 19-May-2025 ]}