ThomasPointProcess
ThomasPointProcess[μ,λ,σ,d]
表示 ![]() 中的一个托马斯聚类点过程,其密度为 μ,聚类平均值为 λ,尺度参数为 σ.
中的一个托马斯聚类点过程,其密度为 μ,聚类平均值为 λ,尺度参数为 σ.
更多信息

- ThomasPointProcess 模拟聚类点布局,其中心均匀地分布在空间上,聚类点以轻尾径向分布方式各向同性地排布在空间中.
-
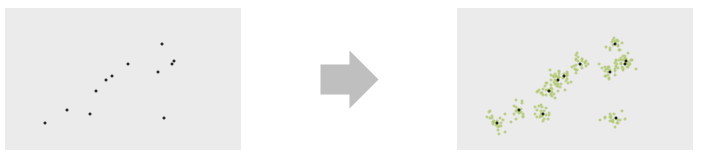
- 常见用途包括宇宙学(其中聚类是星系团)或植物生态学等领域.
- 聚类中心按密度为 μ 的 PoissonPointProcess 分布.
- 聚类的点数按均值为 λ 的 PoissonDistribution 分布.
- 每个聚类中的聚类点按以聚类中心为中心的径向分布 NormalDistribution[0,σ] 各向同性地排布在空间中.
-
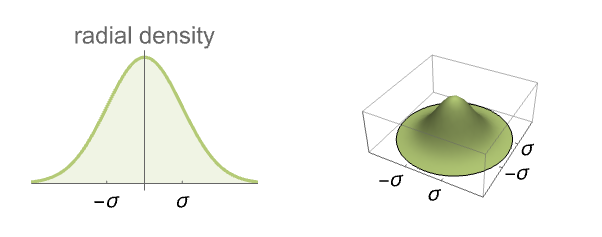
- ThomasPointProcess 允许 μ、λ、和 σ 为任意正实数,允许 d 为任意正整数.
- PointProcessEstimator 可使用以下设置来估计 ThomasPointProcess:
-
"FindClusters" 使用 FindClusters 函数 "MethodOfMoments" 使用同质性度量来估计参数 - ThomasPointProcess 可与诸如 RipleyK、PointCountDistribution 和 RandomPointConfiguration 这样的函数一起使用.
范例
打开所有单元关闭所有单元基本范例 (4)
范围 (2)
来自任何有效 RegionQ(其 RegionEmbeddingDimension 等于它的 RegionDimension)的样本:
属性和关系 (5)
PointCountDistribution 是已知的:
二维托马斯点过程的 Ripley's ![]() 和 Besag's
和 Besag's ![]() :
:
托马斯点过程的 Ripley's ![]() 比泊松点过程 Ripley 的大:
比泊松点过程 Ripley 的大:
托马斯点过程的 Besag's ![]() 比泊松点过程 Ripley 的大:
比泊松点过程 Ripley 的大:
Wolfram Research (2020),ThomasPointProcess,Wolfram 语言函数,https://reference.wolfram.com/language/ref/ThomasPointProcess.html.
文本
Wolfram Research (2020),ThomasPointProcess,Wolfram 语言函数,https://reference.wolfram.com/language/ref/ThomasPointProcess.html.
CMS
Wolfram 语言. 2020. "ThomasPointProcess." Wolfram 语言与系统参考资料中心. Wolfram Research. https://reference.wolfram.com/language/ref/ThomasPointProcess.html.
APA
Wolfram 语言. (2020). ThomasPointProcess. Wolfram 语言与系统参考资料中心. 追溯自 https://reference.wolfram.com/language/ref/ThomasPointProcess.html 年