FrechetDistribution

FrechetDistribution[α,β]

represents the Fréchet distribution with shape parameter α and scale parameter β.

FrechetDistribution[α,β,μ]

represents the Fréchet distribution with shape parameter α, scale parameter β, and location parameter μ.

Details

  • The Fréchet distribution gives the asymptotic distribution of the maximum value in a sample from a distribution such as the Cauchy distribution.
  • FrechetDistribution is also known as type II extreme value distribution.
  • The probability density for value 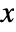in a Fréchet distribution is proportional to 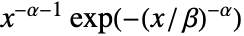for 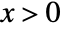and zero otherwise.
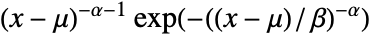  • The probability density for value in a Fréchet distribution with location parameter is proportional to for 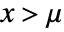and zero otherwise.
  • FrechetDistribution allows α and β to be any positive real numbers and μ to be any real number.
  • FrechetDistribution allows β and μ to be any quantities with the same unit dimensions and α to be a dimensionless quantity. »
  • FrechetDistribution can be used with such functions as Mean, CDF, and RandomVariate.

Background & Context

  • FrechetDistribution[α,β,μ] represents a continuous statistical distribution defined over the interval 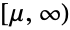and parametrized by a real number μ (called a "location parameter") and two positive real numbers α and β (called a "shape parameter" and a "scale parameter", respectively). The probability density function (PDF) of a Fréchet distribution is unimodal, with the parameter μ determining the horizontal offset of the PDF and the parameters α and β together determining the PDF's overall height and steepness. In addition, the tails of the PDF are "thin", in the sense that the PDF decreases exponentially for large values of 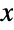. (This behavior can be made quantitatively precise by analyzing the SurvivalFunction of the distribution.) The Fréchet distribution is sometimes referred to as a type II extreme value distribution (not to be confused with "the" extreme value distribution, implemented in the Wolfram Language as ExtremeValueDistribution).
  • FrechetDistribution is one of four distributions (along with GumbelDistribution, ExtremeValueDistribution, and WeibullDistribution) classified under the general heading "extreme value distributions" and is therefore used as a tool for quantifying "extreme" or "rare" events. Named for French mathematician Maurice René Fréchet, the Fréchet distribution was introduced in the late 1920s as a possible limit distribution for the largest order statistic (i.e. as a potential asymptotic distribution for the maximum value of a sample distributed according to some other distribution). Since its inception, the Fréchet distribution has been linked to the modeling of a number of real-world phenomena, including human lifetimes, radioactive emissions, flood and seismic analyses, and maximum one-day rainfalls.
  • RandomVariate can be used to give one or more machine- or arbitrary-precision (the latter via the WorkingPrecision option) pseudorandom variates from a Fréchet distribution. Distributed[x,FrechetDistribution[α,β,μ]], written more concisely as xFrechetDistribution[α,β,μ], can be used to assert that a random variable x is distributed according to a Fréchet distribution. Such an assertion can then be used in functions such as Probability, NProbability, Expectation, and NExpectation.
  • The probability density and cumulative distribution functions may be given using PDF[FrechetDistribution[α,β,μ],x] and CDF[FrechetDistribution[α,β,μ],x]. The mean, median, variance, raw moments, and central moments may be computed using Mean, Median, Variance, Moment, and CentralMoment, respectively.
  • DistributionFitTest can be used to test if a given dataset is consistent with a Fréchet distribution, EstimatedDistribution to estimate a Fréchet parametric distribution from given data, and FindDistributionParameters to fit data to a Fréchet distribution. ProbabilityPlot can be used to generate a plot of the CDF of given data against the CDF of a symbolic Fréchet distribution and QuantilePlot to generate a plot of the quantiles of given data against the quantiles of a symbolic Fréchet distribution.
  • TransformedDistribution can be used to represent a transformed Fréchet distribution, CensoredDistribution to represent the distribution of values censored between upper and lower values, and TruncatedDistribution to represent the distribution of values truncated between upper and lower values. CopulaDistribution can be used to build higher-dimensional distributions that contain a Fréchet distribution, and ProductDistribution can be used to compute a joint distribution with independent component distributions involving Fréchet distributions.
  • The Fréchet distribution is related to several other distributions. As mentioned previously, FrechetDistribution shares qualitative relationships with ExtremeValueDistribution, GumbelDistribution, and WeibullDistribution. These relationships can be quantified by noting that the PDF of FrechetDistribution[α,β] is precisely the same as TransformedDistribution[β2/z,zWeibullDistribution[α,β]], and that WeibullDistribution can be written as transformed versions of both ExtremeValueDistribution and GumbelDistribution. The PDF of FrechetDistribution[α,β] is the same as MaxStableDistribution[β,β/α,1/α] and MinStableDistribution[-β,β/α,1/α] under suitable transformations and assumptions and can be obtained from UniformDistribution under a suitable chain of transformations. FrechetDistribution is also related to ExpGammaDistribution, ExponentialDistribution, and LogisticDistribution.

Examples

open allclose all

Basic Examples  (5)

Probability density function:

With location parameter:

Cumulative distribution function:

With location parameter:

Mean:

Variance:

Median:

Scope  (8)

Generate a sample of pseudorandom numbers from a Fréchet distribution:

Compare its histogram to the PDF:

Distribution parameters estimation:

Estimate the distribution parameters from sample data:

Compare the density histogram of the sample with the PDF of the estimated distribution:

Skewness depends only on the first parameter:

Limiting values:

Kurtosis depends only on the first parameter:

Limiting values:

Different moments with closed forms as functions of parameters:

Moment:

Closed form for symbolic order:

CentralMoment:

FactorialMoment:

Cumulant:

Hazard function:

With location parameter:

Quantile function:

With location parameter:

Consistent use of Quantity in parameters yields QuantityDistribution:

Convert to milliliters:

Find the median:

Applications  (2)

According to a study, the annual maximal tephra (solid material) volume in volcanic eruptions follows a FrechetDistribution with shape parameter 0.71 and scale parameter 6.3, given in cubic kilometers:

Find median annual maximal tephra volume:

Find the average annual maximal tephra volume:

Find the mode of the distribution:

Find the probability that the annual maximal tephra volume is greater than 30 cubic kilometers:

Simulate the annual maximal tephra volume for the next 30 years:

FrechetDistribution can be used to model annual maximum wind speeds:

Fit the distribution to the data:

Compare the histogram of the data with the PDF of the estimated distribution:

Find the probability of annual maximum wind exceeding 90 km/h:

Find average annual maximum wind speed:

Simulate maximum wind speed for 30 years:

Properties & Relations  (8)

Fréchet distribution is closed under positive parameter scaling and translation:

The family of FrechetDistribution is closed under maximum:

CDF of FrechetDistribution solves the maximum stability postulate equation:

Fix and and simplify:

Relationships to other distributions:

The default location is 0:

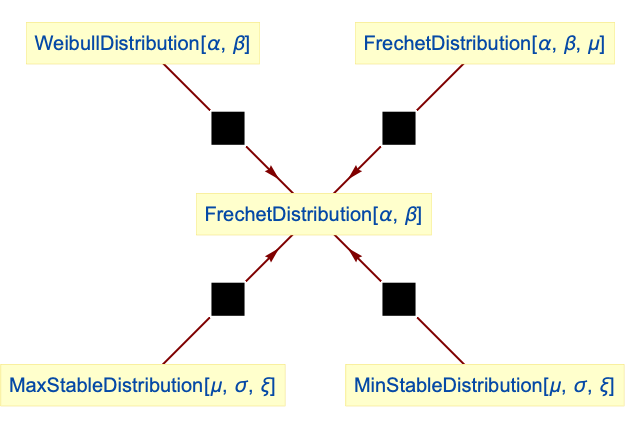
Fréchet distribution is a transformation of WeibullDistribution:

Fréchet distribution is related to MaxStableDistribution:

Fréchet distribution is related to MinStableDistribution:

Neat Examples  (1)

PDFs for different α values with CDF contours:

Wolfram Research (2010), FrechetDistribution, Wolfram Language function, https://reference.wolfram.com/language/ref/FrechetDistribution.html (updated 2016).

Text

Wolfram Research (2010), FrechetDistribution, Wolfram Language function, https://reference.wolfram.com/language/ref/FrechetDistribution.html (updated 2016).

CMS

Wolfram Language. 2010. "FrechetDistribution." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2016. https://reference.wolfram.com/language/ref/FrechetDistribution.html.

APA

Wolfram Language. (2010). FrechetDistribution. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/FrechetDistribution.html

BibTeX

@misc{reference.wolfram_2025_frechetdistribution, author="Wolfram Research", title="{FrechetDistribution}", year="2016", howpublished="\url{https://reference.wolfram.com/language/ref/FrechetDistribution.html}", note=[Accessed: 09-May-2025 ]}

BibLaTeX

@online{reference.wolfram_2025_frechetdistribution, organization={Wolfram Research}, title={FrechetDistribution}, year={2016}, url={https://reference.wolfram.com/language/ref/FrechetDistribution.html}, note=[Accessed: 09-May-2025 ]}