Variance
Variance[data]
gives the variance estimate of the elements in data.
Variance[dist]
gives the variance of the distribution dist.
Details
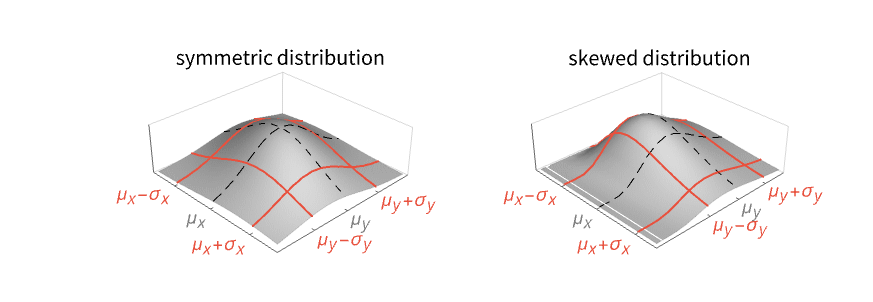
- Variance measures dispersion of data or distributions.
- Variance[data] gives the unbiased estimate of variance.
- For vector data
, the variance estimate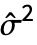 
is given by 
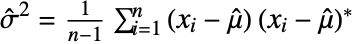for reals and 
for complexes and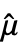 
=Mean[data]. -
- For matrix data, the variance estimate
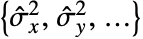is computed for each column vector, with Variance[{{x1,y1,…},{x2,y2,…},…}] equivalent to {Variance[{x1,x2,…}],Variance[{y1,y2,…}]}. » -
- For array data, variance is equivalent to ArrayReduce[Variance,data,1]. »
-
↓ ↓ ↓ ↓ ↓ 
- For a real weighted WeightedData[{x1,x2,…},{w1,w2,…}], the variance is given by
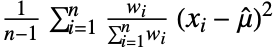. » - Variance handles both numerical and symbolic data.
- The data can have the following additional forms and interpretations:
-
Association the values (the keys are ignored) » SparseArray as an array, equivalent to Normal[data] » QuantityArray quantities as an array » WeightedData weighted variance, based on the underlying EmpiricalDistribution » EventData based on the underlying SurvivalDistribution » TimeSeries, TemporalData, … vector or array of values (the time stamps ignored) » Image,Image3D RGB channel's values or grayscale intensity value » Audio amplitude values of all channels » - For a univariate distribution dist, the variance is given by σ2=Expectation[(x-μ)2,xdist] with μ=Mean[dist]. »
-
- For a multivariate distribution dist, the variance is given by {σx2,σy2,…}=Expectation[{(x-μx)2,(y-μy)2,…},{x,y,…}dist]. »
-
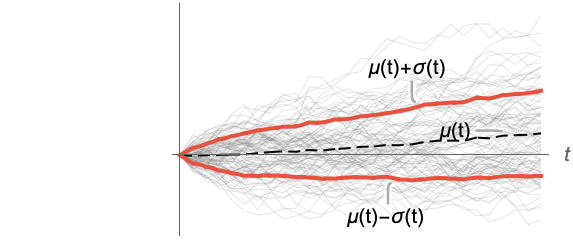
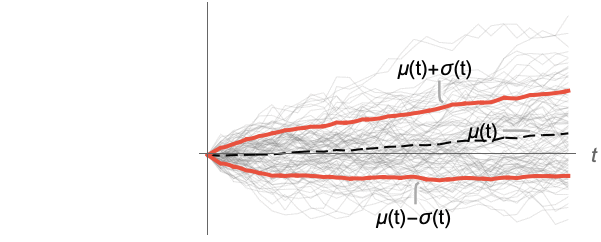
- For a random process proc, the variance function can be computed for slice distribution at time t, SliceDistribution[proc,t], as σ[t]2=Variance[SliceDistribution[proc,t]]. »
-
Examples
open allclose allBasic Examples (3)
Scope (17)
Basic Uses (7)
Exact input yields exact output:
Approximate input yields approximate output:
Find the variance of WeightedData:
Find the variance of EventData:
Find the variance of TemporalData:
Find the variance of a TimeSeries:
Array Data (5)
Variance for a matrix gives columnwise variances:
Variance for a tensor gives columnwise variances at the first level:
When the input is an Association, Variance works on its values:
SparseArray data can be used just like dense arrays:
Find the variance of a QuantityArray:
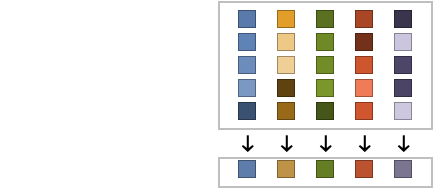
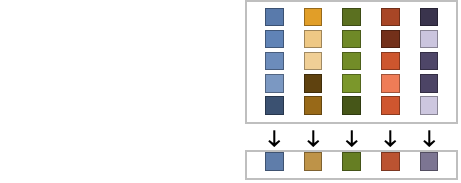
Image and Audio Data (2)
Channelwise variance of an RGB image:
Variance of a grayscale image:
On audio objects, Variance works channelwise:
Applications (5)
Variance is a measure of dispersion:
Compute a moving variance for samples of three random processes:
Compare data volatility by smoothing with moving variance:
Find the mean and variance for the number of great inventions and scientific discoveries in each year from 1860 to 1959:
Investigate weak stationarity of the process data by analyzing variance of slices:
Use a larger plot range to see how relatively small the variations are:
Find the variance of the heights for the children in a class:
Properties & Relations (11)
The square root of Variance is StandardDeviation:
Variance is a scaled squared Norm of deviations from the Mean:
Variance is a scaled CentralMoment:
The square root of Variance is a scaled RootMeanSquare of the deviations:
Variance is a scaled Mean of squared deviations from the Mean:
Variance is a scaled SquaredEuclideanDistance from the Mean:
Variance is less than MeanDeviation if all absolute deviations are less than 1:
Variance is greater than MeanDeviation if all absolute deviations are greater than 1:
Variance of a random variable as an Expectation:
Variance gives an unbiased sample estimate:
Unbiased means that the expected value of the sample variance with respect to the population distribution equals the variance of the underlying distribution:
Variance gives an unbiased weighted sample estimate:
Unbiased means that the expected value of the sample variance with respect to the population distribution equals the variance of the underlying distribution:
Neat Examples (1)
The distribution of Variance estimates for 20, 100, and 300 samples:
Text
Wolfram Research (2003), Variance, Wolfram Language function, https://reference.wolfram.com/language/ref/Variance.html (updated 2023).
CMS
Wolfram Language. 2003. "Variance." Wolfram Language & System Documentation Center. Wolfram Research. Last Modified 2023. https://reference.wolfram.com/language/ref/Variance.html.
APA
Wolfram Language. (2003). Variance. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/Variance.html