CircularOrthogonalMatrixDistribution
CircularOrthogonalMatrixDistribution[n]
行列次元が{n,n}の円直交行列分布を表す.
詳細
- CircularOrthogonalMatrixDistributionは,円直交アンサンブル(COE)としても知られている.
- CircularOrthogonalMatrixDistributionは,ユニタリ群
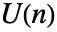 の対称部分集合上のHaar測度としても知られる,次元 n の対称ユニタリ正方行列上の一様分布を表す.
の対称部分集合上のHaar測度としても知られる,次元 n の対称ユニタリ正方行列上の一様分布を表す. - 次元母数 n は任意の正の整数でよい.
- CircularOrthogonalMatrixDistributionは,MatrixPropertyDistributionやRandomVariate等の関数とともに使うことができる.
予備知識
- CircularOrthogonalMatrixDistribution[n]は,円直交アンサンブル(COE)とも呼ばれるもので,
 ユニタリ行列および対称複素行列上の,具体的には
ユニタリ行列および対称複素行列上の,具体的には  と
と 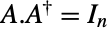 の両方を満足する複素正方行列
の両方を満足する複素正方行列 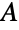 上の統計分布を表す.ただし,
上の統計分布を表す.ただし,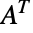 は
は 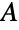 の転置を,
の転置を,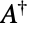 は
は  の共役転置を,
の共役転置を,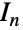 は
は 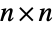 恒等行列を表す.母数 n は分布の次元母数と呼ばれるもので,任意の正の整数でよい.「円直交行列分布」という名前ではあるが,この分布に属する行列はユニタリ行列(
恒等行列を表す.母数 n は分布の次元母数と呼ばれるもので,任意の正の整数でよい.「円直交行列分布」という名前ではあるが,この分布に属する行列はユニタリ行列(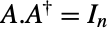 )だが,必ずしも直交(
)だが,必ずしも直交(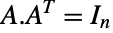 )ではない.
)ではない. - 円直交行列分布は,円シンプレクティック行列分布(CircularSymplecticMatrixDistribution)および円ユニタリ行列分布(CircularUnitaryMatrixDistribution)と並んで,Freeman Dysonが量子力学の研究のために1962年に考案した3つの円行列アンサンブルの一つである.円直交行列分布は,確率的には対称ユニタリ正方行列の集合上の一様分布を表すが,数学的にはユニタリ群
 内のすべての対称行列の部分集合上のいわゆるハール(Haar)測度である.円直交行列分布のような行列アンサンブルは,ランダム行列理論をはじめとする物理学および数学のさまざま分野の研究において非常に重要である.
内のすべての対称行列の部分集合上のいわゆるハール(Haar)測度である.円直交行列分布のような行列アンサンブルは,ランダム行列理論をはじめとする物理学および数学のさまざま分野の研究において非常に重要である. - RandomVariateを使って,円直交行列分布から,1つあるいは複数の機械精度あるいは任意精度(後者はWorkingPrecisionオプションを介す)の擬似乱数変量を得ることができる.そのような変量の集合の平均,中央値,分散,モーメント,中心モーメントは,それぞれMean,Median,Variance,Moment,CentralMomentを使って計算することができる.Distributed[A,CircularOrthogonalMatrixDistribution[n]](より簡単な表記では ACircularOrthogonalMatrixDistribution[n])を使ってランダム行列 A が円直交行列分布に従って分布していると宣言することができる.そのような宣言はMatrixPropertyDistribution等の関数で使うことができる.
- 円直交行列分布に従って分布する変量のトレース,固有値,ノルムは,それぞれTr,Eigenvalues,Normを使って計算することができる.そのような変量は,MatrixFunctionやMatrixPowerで調べることもできる.加えて,実部(Re),虚部(Im),複素引数(Arg) 等の関連する実際の量はMatrixPlotを使ってプロットできる.
- CircularOrthogonalMatrixDistributionは,他の多くの分布と関係がある.上述の通り,この分布はCircularQuaternionMatrixDistribution,CircularRealMatrixDistribution,CircularSymplecticMatrixDistribution,CircularUnitaryMatrixDistribution等の他の円形行列分布と定性的に類似している.円行列アンサンブルは,もともとはいわゆるガウスアンサンブルの一般化として導かれたものなので,CircularOrthogonalMatrixDistributionはGaussianOrthogonalMatrixDistribution,GaussianSymplecticMatrixDistribution,GaussianUnitaryMatrixDistributionとも関係がある.CircularOrthogonalMatrixDistributionはMatrixNormalDistribution,MatrixTDistribution,WishartMatrixDistribution,InverseWishartMatrixDistribution,TracyWidomDistribution,WignerSemicircleDistributionとも関係がある.
例題
すべて開くすべて閉じる例 (2)
MatrixPropertyDistributionを使って球上のランダムな点をサンプルする:
アプリケーション (2)
結合位相分布を閉形式のPDFとともに可視化する:
CircularOrthogonalMatrixDistributionの固有値の結合分布は,逆温度が ![]() の,円上のDysonのCoulombガスのBoltzmann分布としても知られている.系の粒子あたりの平均ハミルトニアンは以下の通りである(運動項は除く):
の,円上のDysonのCoulombガスのBoltzmann分布としても知られている.系の粒子あたりの平均ハミルトニアンは以下の通りである(運動項は除く):
ランダムな円直交アンサンブル行列のハミルトニアンの値の分布を定義する:
特性と関係 (2)
サンプルレベルの間隔のヒストグラムを,Dyson指数 ![]() のWigner推測としても知られる閉形式と比較する:
のWigner推測としても知られる閉形式と比較する:
次元 ![]() 大のCircularOrthogonalMatrixDistributionの固有値については,要素のスケールされた法はカイ二乗分布に従う:
大のCircularOrthogonalMatrixDistributionの固有値については,要素のスケールされた法はカイ二乗分布に従う:
ヒストグラムをChiSquareDistributionのPDFと比較する:
考えられる問題 (2)
CircularOrthogonalMatrixDistributionの行列形式は直交行列である必要はない:
CircularRealMatrixDistributionを使ってランダム直交実数値行列をサンプルする:
CircularOrthogonalMatrixDistributionの行列形式は行列 ![]() で表すことができる.ただし,
で表すことができる.ただし,![]() はCircularUnitaryMatrixDistributionに従う:
はCircularUnitaryMatrixDistributionに従う:
テキスト
Wolfram Research (2015), CircularOrthogonalMatrixDistribution, Wolfram言語関数, https://reference.wolfram.com/language/ref/CircularOrthogonalMatrixDistribution.html.
CMS
Wolfram Language. 2015. "CircularOrthogonalMatrixDistribution." Wolfram Language & System Documentation Center. Wolfram Research. https://reference.wolfram.com/language/ref/CircularOrthogonalMatrixDistribution.html.
APA
Wolfram Language. (2015). CircularOrthogonalMatrixDistribution. Wolfram Language & System Documentation Center. Retrieved from https://reference.wolfram.com/language/ref/CircularOrthogonalMatrixDistribution.html