DiggleGatesPointProcess
DiggleGatesPointProcess[μ,ρ,d]
表示一个 Diggle–Gates 点过程,其固定强度为 μ 且交互半径为 ![]() 中的 ρ.
中的 ρ.
更多信息


- DiggleGatesPointProcess 模拟点布局,其中的点在相互距离半径 ρ 的范围内有一个软核递减互斥配对作用,否则均匀分布.
-
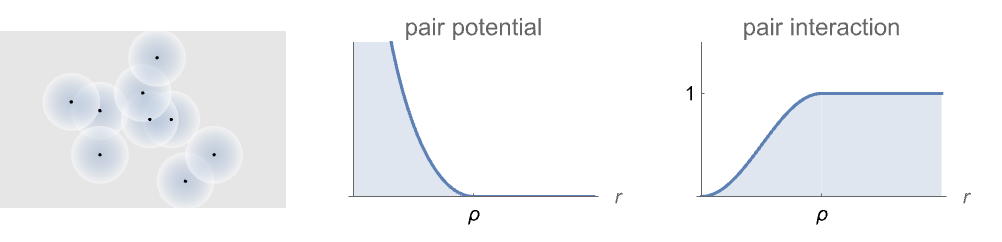
- Diggle–Gates 点过程可定义为关于其强度 μ 和配对势能
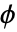 或配对作用
或配对作用 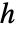 的 GibbsPointProcess,皆可通过 ρ 按照下列方式参数化:
的 GibbsPointProcess,皆可通过 ρ 按照下列方式参数化: -
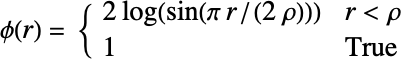
配对势能 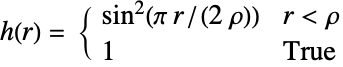
配对作用 - 在区域 reg 中,Diggle–Gates 点过程 DiggleGatesPointProcess[μ,ρ,d] 中的点布局
 的关于 PoissonPointProcess[1,d] 的密度函数
的关于 PoissonPointProcess[1,d] 的密度函数  与
与 ![mu^n product_(i!=j)h(TemplateBox[{{{p, _, i}, -, {p, _, j}}}, Norm]) mu^n product_(i!=j)h(TemplateBox[{{{p, _, i}, -, {p, _, j}}}, Norm])](Files/DiggleGatesPointProcess.zh/9.png) 成比例.
成比例. - 将点
 添加到点布局
添加到点布局  的 Papangelou 条件密度
的 Papangelou 条件密度 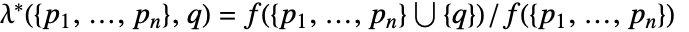 为
为 ![mu product_ih(TemplateBox[{{{p, _, i}, -, q}}, Norm]) mu product_ih(TemplateBox[{{{p, _, i}, -, q}}, Norm])](Files/DiggleGatesPointProcess.zh/13.png) .
. - DiggleGatesPointProcess 允许 μ 和 ρ 为任意正数,且 d 为任意正整数.
- DiggleGatesPointProcess 是 GibbsPointProcess 的特殊情况.
- 当
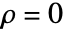 时,DiggleGatesPointProcess 简化为 PoissonPointProcess.
时,DiggleGatesPointProcess 简化为 PoissonPointProcess. - RandomPointConfiguration 中,StraussPointProcess 的可能 Method 设置为:
-
"MCMC" 马尔科夫链蒙特卡洛方法初始和消亡 "Exact" 从过去耦合 - EstimatedPointProcess 中,DiggleGatesPointProcess 可能的 PointProcessEstimator 设置为:
-
Automatic 自动选择参数估计量 "MaximumPseudoLikelihood" 最大化伪似然度 - DiggleGatesPointProcess 可与诸如 RipleyK 和 RandomPointConfiguration 这样的函数一起使用.
范例
打开所有单元关闭所有单元
Wolfram Research (2020),DiggleGatesPointProcess,Wolfram 语言函数,https://reference.wolfram.com/language/ref/DiggleGatesPointProcess.html.
文本
Wolfram Research (2020),DiggleGatesPointProcess,Wolfram 语言函数,https://reference.wolfram.com/language/ref/DiggleGatesPointProcess.html.
CMS
Wolfram 语言. 2020. "DiggleGatesPointProcess." Wolfram 语言与系统参考资料中心. Wolfram Research. https://reference.wolfram.com/language/ref/DiggleGatesPointProcess.html.
APA
Wolfram 语言. (2020). DiggleGatesPointProcess. Wolfram 语言与系统参考资料中心. 追溯自 https://reference.wolfram.com/language/ref/DiggleGatesPointProcess.html 年